9.2.3

Black Body Radiation

Test yourself on Black Body Radiation

Test your knowledge with free interactive questions on Seneca — used by over 10 million students.

Black Body Radiation

Stars can be modelled very accurately as black bodies.

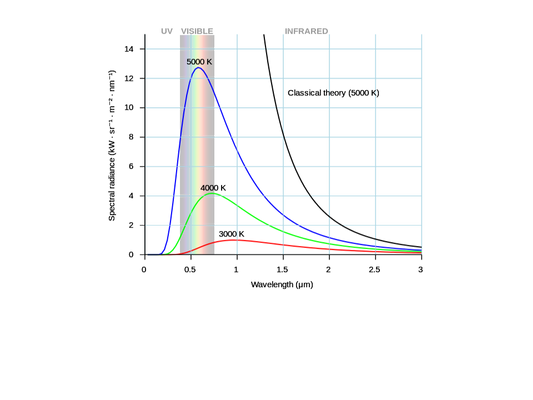
Black body

Black body

  • A black body is defined as the universal emitter.
  • At a given temperature, the intensity of light at each wavelength follows the curve shown in the diagram.
  • Stars are assumed to be black bodies.
Temperature and wavelength

Temperature and wavelength

  • The diagram also shows that stars of different temperatures have different distributions, characterised by the wavelength of maximum intensity.
Colour

Colour

  • A star’s colour is related to this distribution:
    • Cool stars are more red in colour.
    • Warmer stars are white as they emit roughly equally across the visible spectrum.
    • Very hot stars appear blue.

Stefan's Law

Stefan’s Law and Wien’s Displacement Law are two ideas that assist in measuring and comparing the power outputs, temperatures and sizes of stars.

Stefan's law

Stefan's law

  • Power output of star:
    • P=σ×A×T4P=\sigma \times A \times T^4
  • Where T is the temperature (in Kelvin) of the surface of the star, A is the surface area of the star and σ (sigma) is Stefan’s constant.
Find size of star

Find size of star

  • If we know the temperature and power emitted by a star, we can work out its surface area, A, and therefore its radius (assuming the star is a perfect sphere).

Wien's Displacement Law

Wien’s Displacement law describes the relationship between the wavelength of maximum intensity, λ, and T, the surface temperature of the star (in Kelvin).

Wien's displacement law

Wien's displacement law

  • The wavelength at which the star emits light most intensely:
  • λmax×T=constant=2.9×10−3\lambda_{max}\times T= constant = 2.9\times 10^{-3}mK
Application

Application

  • Astronomers can measure the intensity of light from a star at the Earth and the distance of the star from the Earth.
  • Wien’s displacement law allows an estimate of the temperature of the star to be made.
  • Stefan’s Law can then be used to estimate the radius of the star.
Jump to other topics
1

Measurements & Errors

1.1

Measurements & Errors

1.1.1SI Base Units1.1.2Combining SI Units1.1.3Prefixes of Units1.1.4Standard Form1.1.5Converting Units1.1.6End of Topic Test - Units & Prefixes1.1.7Limitation of Physical Measurements1.1.8Uncertainty1.1.9Estimation1.1.10End of Topic Test - Measurements & Errors1.1.11Diagnostic Misconceptions - Converting between SI1.1.12Diagnostic Misconceptions - SI & Area/Volume
2

Particles & Radiation

2.1

Particles

2.1.1Atomic Model2.1.2Specific Charge, Protons & Neutron Numbers2.1.3End of Topic Test - Atomic Model2.1.4Isotopes2.1.5Stable & Unstable Nuclei2.1.6End of Topic Test - Isotopes & Nuclei2.1.7A-A* (AO3/4) - Stable & Unstable Nuclei2.1.8Particles, Antiparticles & Photons2.1.9Particle Interactions2.1.10Classification of Particles2.1.11End of Topic Test - Particles & Interactions2.1.12Quarks & Antiquarks2.1.13Application of Conservation Laws2.1.14End of Topic Test - Leptons & Quarks2.1.15Exam-Style Question - Radioactive Decay
2.2

Electromagnetic Radiation & Quantum Phenomena

2.2.1The Photoelectric Effect2.2.2The Photoelectric Effect Explanation2.2.3End of Topic Test - The Photoelectric Effect2.2.4Collisions of Electrons with Atoms2.2.5Energy Levels & Photon Emission2.2.6Wave-Particle Duality2.2.7End of Topic Test - Absorption & Emission2.2.8Diagnostic Misconceptions - Electron Volts2.2.9Diagnostic Misconceptions - Converting eV & Joules
3

Waves

3.1

Progressive & Stationary Waves

3.1.1Progressive Waves3.1.2Wave Speed & Phase Difference3.1.3Longitudinal & Transverse Waves3.1.4End of Topic Test - Progressive Waves3.1.5Polarisation3.1.6Stationary Waves3.1.7Stationary Waves 23.1.8End of Topic Test - Polarisation & Stationary Wave3.1.9A-A* (AO3/4) - Stationary Waves
3.2

Refraction, Diffraction & Interference

3.2.1Interference3.2.2Interference 23.2.3End of Topic Test - Interference3.2.4Diffraction3.2.5Diffraction Gratings3.2.6End of Topic Test - Diffraction3.2.7Refraction at a Plane Surface3.2.8Internal Reflection & Fibre Optics3.2.9End of Topic Test - Refraction3.2.10Exam-Style Question - Waves
4

Mechanics & Materials

4.1

Force, Energy & Momentum

4.1.1Scalars & Vectors4.1.2Vector Problems4.1.3End of Topic Test - Scalars & Vectors4.1.4Moments4.1.5Centre of Mass4.1.6End of Topic Test - Moments & Centre of Mass4.1.7Motion in a Straight Line4.1.8Graphs of Motion4.1.9Bouncing Ball Example4.1.10End of Topic Test - Motion in a Straight Line4.1.11Acceleration Due to Gravity4.1.12Projectile Motion4.1.13Friction4.1.14Terminal Speed4.1.15End of Topic Test - Acceleration Due to Gravity4.1.16Newton's Laws4.1.17Momentum4.1.18Momentum 24.1.19End of Topic Test - Newton's Laws & Momentum4.1.20A-A* (AO3/4) - Newton's Third Law4.1.21Work & Energy4.1.22Power & Efficiency4.1.23Conservation of Energy4.1.24End of Topic Test - Work, Energy & Power4.1.25Exam-Style Question - Forces4.1.26Diagnostic Misconceptions - Perpendicular Vectors4.1.27Diagnostic Misconceptions - Weight Acts Downwards4.1.28Diagnostic Misconceptions - Acceleration Direction4.1.29Diagnostic Misconceptions - Stationary Objects4.1.30Diagnostic Misconceptions - Action & Reaction4.1.31Diagnostic Misconceptions - RF Direction4.1.32Diagnostic Misconceptions - RF Acceleration4.1.33Diagnostic Misconceptions - Change in Momentum
4.2

Materials

4.2.1Density4.2.2Bulk Properties of Solids4.2.3Energy in Materials4.2.4Young Modulus4.2.5End of Topic Test - Materials
5

Electricity

5.1

Current Electricity

5.1.1Basics of Electricity5.1.2Current-Voltage Characteristics5.1.3End of Topic Test - Basics of Electricity5.1.4Resistivity5.1.5Superconductivity5.1.6A-A* (AO3/4) - Superconductivity5.1.7End of Topic Test - Resistivity & Superconductors5.1.8Circuits5.1.9Power and Conservation5.1.10Potential Divider5.1.11Emf & Internal Resistance5.1.12End of Topic Test - Power & Potential5.1.13Exam-Style Question - Resistance5.1.14Diagnostic Misconceptions - Constant Current5.1.15Diagnostic Misconceptions - Potential Difference
6

Further Mechanics & Thermal Physics (A2 only)

6.1

Periodic Motion (A2 only)

6.1.1Circular Motion6.1.2Circular Motion 26.1.3End of Topic Test - Circular Motion6.1.4Simple Harmonic Motion6.1.5Simple Harmonic Systems6.1.6Energy in Simple Harmonic Motion6.1.7Resonance6.1.8End of Topic Test - Simple Harmonic Motion6.1.9A-A* (AO3/4) - Simple Harmonic Motion
6.2

Thermal Physics (A2 only)

6.2.1Thermal Energy Transfer6.2.2Thermal Energy Transfer Experiments6.2.3Ideal Gases6.2.4Ideal Gases 26.2.5Boyle's Law & Charles' Law6.2.6Molecular Kinetic Theory Model6.2.7Molecular Kinetic Theory Model 26.2.8End of Topic Test - Thermal Energy & Ideal Gases6.2.9Exam-Style Question - Ideal Gases6.2.10Diagnostic Misconceptions - Material & Mass6.2.11Diagnostic Misconceptions - No Energy
7

Fields & Their Consequences (A2 only)

7.1

Fields (A2 only)

7.1.1Fields
7.2

Gravitational Fields (A2 only)

7.2.1Newton's Law7.2.2Gravitational Field Strength7.2.3Gravitational Potential7.2.4Orbits of Planets & Satellites7.2.5Escape Velocity & Synchronous Orbits7.2.6End of Topic Test - Gravitational Fields
7.3

Electric Fields (A2 only)

7.3.1Coulomb's Law7.3.2Electric Field Strength7.3.3Electric Field Strength 27.3.4Electric Potential7.3.5End of Topic Test - Electric Fields7.3.6A-A* (AO3/4) - Electric and Gravitational Field
7.4

Capacitance (A2 only)

7.4.1Capacitance7.4.2Parallel Plate Capacitor7.4.3Energy Stored by a Capacitor7.4.4Capacitor Discharge7.4.5Capacitor Charge
7.5

Magnetic Fields (A2 only)

7.5.1Magnetic Flux Density7.5.2End of Topic Test - Capacitance & Flux Density7.5.3Moving Charges in a Magnetic Field7.5.4Magnetic Flux & Flux Linkage7.5.5Electromagnetic Induction7.5.6Electromagnetic Induction 27.5.7Alternating Currents7.5.8Operation of a Transformer7.5.9Magnetic Flux Density7.5.10End of Topic Test - Electromagnetic Induction
8

Nuclear Physics (A2 only)

8.1

Radioactivity (A2 only)

8.1.1Rutherford Scattering8.1.2Alpha & Beta Radiation8.1.3Gamma Radiation8.1.4Radioactive Decay8.1.5Half Life8.1.6End of Topic Test - Radioactivity8.1.7Nuclear Instability8.1.8Nuclear Radius8.1.9Mass & Energy8.1.10Binding Energy8.1.11Induced Fission8.1.12Safety Aspects of Nuclear Reactors8.1.13End of Topic Test - Nuclear Physics8.1.14A-A* (AO3/4) - Nuclear Fusion
9

Option: Astrophysics (A2 only)

9.1

Telescopes (A2 only)

9.1.1Astronomical Telescopes9.1.2Reflecting Telescopes9.1.3Single Dish Radio Telescopes9.1.4Large Diameter Telescopes
9.2

Classification of Stars (A2 only)

9.2.1Classification by Luminosity9.2.2Absolute Magnitude9.2.3Black Body Radiation9.2.4Stellar Spectral Classes9.2.5Hertzsprung-Russell Diagrams9.2.6Astronomical Objects
9.3

Cosmology (A2 only)

9.3.1Doppler Effect9.3.2Hubble's Law9.3.3Quasars9.3.4Detecting Exoplanets
10

Option: Medical Physics (A2 only)

10.1

Physics of the Eye (A2 only)

10.1.1Physics of Vision10.1.2Defects of Vision10.1.3Lenses10.1.4Correcting Defects of Vision
10.2

Physics of the Ear (A2 only)

10.2.1Structure of the Ear10.2.2Sensitivity of the Ear10.2.3Hearing Defects
10.3

Biological Measurement (A2 only)

10.3.1Electrocardiography (ECG)
10.4

Non-Ionising Imaging (A2 only)

10.4.1Ultrasound Imaging10.4.2Ultrasound Imaging 210.4.3Fibre Optics & Endoscopy10.4.4Magnetic Resonance Scanning
10.5

X-Ray Imaging (A2 only)

10.5.1Diagnostic X-Rays10.5.2X-Ray Image Processing10.5.3Absorption of X-Rays10.5.4CT Scanners
10.6

Radionuclide Imaging & Therapy (A2 only)

10.6.1Imaging Techniques10.6.2Half Life10.6.3Gamma Camera10.6.4High Energy X-Rays10.6.5Radioactive Implants10.6.6Imaging Comparisons
11

Option: Engineering Physics (A2 only)

11.1

Rotational Dynamics (A2 only)

11.1.1Moment of Inertia11.1.2Rotational Kinetic Energy11.1.3Rotational Motion11.1.4Torque & Angular Acceleration11.1.5Angular Momentum11.1.6Angular Work & Power
11.2

Thermodynamics & Engines (A2 only)

11.2.1First Law of Thermodynamics11.2.2Non-Flow Processes11.2.3p-V Diagrams11.2.4Engine Cycles11.2.5Second Law & Engines11.2.6Reversed Heat Engines
12

Option: Turning Points in Physics (A2 only)

12.1

Discovery of the Electron (A2 only)

12.1.1Cathode Rays12.1.2Thermionic Electron Emission12.1.3Electron Specific Charge12.1.4Millikan's Experiment
12.2

Wave-Particle Duality (A2 only)

12.2.1Newton's & Huygen's Theories of Light12.2.2Electromagnetic Waves12.2.3Photoelectricity12.2.4Wave-Particle Duality12.2.5Electron Microscopes
12.3

Special Relativity (A2 only)

12.3.1Michelson-Morley Experiment12.3.2Einstein's Theory of Special Relativity12.3.3Time Dilation12.3.4Length Contraction12.3.5Mass & Energy

Practice questions on Black Body Radiation

Can you answer these? Test yourself with free interactive practice on Seneca — used by over 10 million students.

  1. 1
    What colour are cool stars?Multiple choice
  2. 2
    Which of these statements are true about star colours?True / false
  3. 3
    Stefan's law is $$P=\sigma A T^4$$. What unit is surface area measured in?Multiple choice
  4. 4
    Stefan's law is $$P=\sigma A T^4$$. What unit is temperature measured in?Multiple choice
  5. 5
    Which of these units are used in Stefan's law?True / false
Answer all questions on Black Body Radiation

Unlock your full potential with Seneca Premium

  • Unlimited access to 10,000+ open-ended exam questions

  • Mini-mock exams based on your study history

  • Unlock 800+ premium courses & e-books

Get started with Seneca Premium

Absolute Magnitude

Stellar Spectral Classes